Difference between revisions of "The Law of Cosines"
| (2 intermediate revisions by the same user not shown) | |||
| Line 2: | Line 2: | ||
[[Image:Law-of-cosines1.svg]] | [[Image:Law-of-cosines1.svg]] | ||
| − | The Pythagorean theorem is a special case of the more general theorem relating the lengths of sides in any triangle, the law of cosines: | + | The Pythagorean theorem is a special case of the more general theorem relating the lengths of sides in any triangle, the law of cosines: |
| − | |||
| − | |||
:<math>a^2+b^2-2ab\cos(\theta)=c^2</math> | :<math>a^2+b^2-2ab\cos(\theta)=c^2</math> | ||
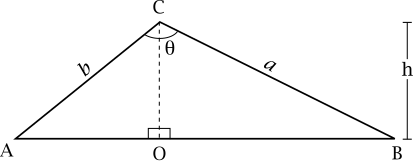
where <math>\theta</math> is the angle between sides <math>a</math> and <math>b</math> . | where <math>\theta</math> is the angle between sides <math>a</math> and <math>b</math> . | ||
| Line 59: | Line 57: | ||
==Resources== | ==Resources== | ||
| − | |||
* [https://mathresearch.utsa.edu/wikiFiles/MAT1093/The%20Law%20of%20Cosines/Esparza%201093%20Notes%204.3A.pdf The Law of Cosines]. Written notes created by Professor Esparza, UTSA. | * [https://mathresearch.utsa.edu/wikiFiles/MAT1093/The%20Law%20of%20Cosines/Esparza%201093%20Notes%204.3A.pdf The Law of Cosines]. Written notes created by Professor Esparza, UTSA. | ||
* [https://mathresearch.utsa.edu/wikiFiles/MAT1093/The%20Law%20of%20Cosines/Esparza%201093%20Notes%204.3B.pdf The Law of Cosines Continued]. Written notes created by Professor Esparza, UTSA. | * [https://mathresearch.utsa.edu/wikiFiles/MAT1093/The%20Law%20of%20Cosines/Esparza%201093%20Notes%204.3B.pdf The Law of Cosines Continued]. Written notes created by Professor Esparza, UTSA. | ||
| + | |||
| + | == Licensing == | ||
| + | Content obtained and/or adapted from: | ||
| + | * [https://en.wikibooks.org/wiki/Trigonometry/Law_of_Cosines Law of Cosines, Wikibooks] under a CC BY-SA license | ||
Latest revision as of 17:00, 28 October 2021
Contents
Law of Cosines
The Pythagorean theorem is a special case of the more general theorem relating the lengths of sides in any triangle, the law of cosines:
where is the angle between sides and .
Does the formula make sense?
This formula had better agree with the Pythagorean Theorem when .
So try it...
When ,
The and the formula reduces to the usual Pythagorean theorem.
Permutations
For any triangle with angles and corresponding opposite side lengths , the Law of Cosines states that
Proof
Dropping a perpendicular from vertex to intersect (or extended) at splits this triangle into two right-angled triangles and , with altitude from side .
First we will find the lengths of the other two sides of triangle in terms of known quantities, using triangle .
Side is split into two segments, with total length .
- has length
- has length
Now we can use the Pythagorean Theorem to find , since .
The corresponding expressions for and can be proved similarly.
The formula can be rearranged:
and similarly for and .
Applications
This formula can be used to find the third side of a triangle if the other two sides and the angle between them are known. The rearranged formula can be used to find the angles of a triangle if all three sides are known.
Resources
- The Law of Cosines. Written notes created by Professor Esparza, UTSA.
- The Law of Cosines Continued. Written notes created by Professor Esparza, UTSA.
Licensing
Content obtained and/or adapted from:
- Law of Cosines, Wikibooks under a CC BY-SA license