Classifying Triangles

The terminology for categorizing triangles is more than two thousand years old, having been defined on the very first page of Euclid's Elements. The names used for modern classification are either a direct transliteration of Euclid's Greek or their Latin translations.

By lengths of sides

Euler diagram of types of triangles, using the definition that isosceles triangles have at least 2 equal sides (i.e., equilateral triangles are isosceles).

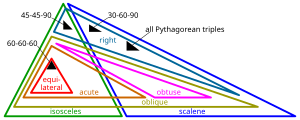
• An equilateral triangle (Greek: ἰσόπλευρον, romanized: isópleuron, lit. 'equal sides') has three sides of the same length. An equilateral triangle is also a regular polygon with all angles measuring 60°.
• An isosceles triangle (Greek: ἰσοσκελὲς, romanized: isoskelés, lit. 'equal legs') has two sides of equal length. An isosceles triangle also has two angles of the same measure, namely the angles opposite to the two sides of the same length. This fact is the content of the isosceles triangle theorem, which was known by Euclid. Some mathematicians define an isosceles triangle to have exactly two equal sides, whereas others define an isosceles triangle as one with at least two equal sides. The latter definition would make all equilateral triangles isosceles triangles. The 45–45–90 right triangle, which appears in the tetrakis square tiling, is isosceles.
• A scalene triangle (Greek: σκαληνὸν, romanized: skalinón, lit. 'unequal') has all its sides of different lengths. Equivalently, it has all angles of different measure.

• 

  Equilateral Triangle

• 

  Isosceles triangle

• 

  Scalene triangle

Hatch marks, also called tick marks, are used in diagrams of triangles and other geometric figures to identify sides of equal lengths. A side can be marked with a pattern of "ticks", short line segments in the form of tally marks; two sides have equal lengths if they are both marked with the same pattern. In a triangle, the pattern is usually no more than 3 ticks. An equilateral triangle has the same pattern on all 3 sides, an isosceles triangle has the same pattern on just 2 sides, and a scalene triangle has different patterns on all sides since no sides are equal.

Similarly, patterns of 1, 2, or 3 concentric arcs inside the angles are used to indicate equal angles: an equilateral triangle has the same pattern on all 3 angles, an isosceles triangle has the same pattern on just 2 angles, and a scalene triangle has different patterns on all angles, since no angles are equal.

By internal angles

The first page of Euclid's Elements, from the world's first printed version (1482), showing the "definitions" section of Book I. The right triangle is labeled "orthogonius", and the two angles shown are "acutus" and "angulus obtusus".

Triangles can also be classified according to their internal angles, measured here in degrees.

• A right triangle (or right-angled triangle, formerly called a rectangled triangle) has one of its interior angles measuring 90° (a right angle). The side opposite to the right angle is the hypotenuse, the longest side of the triangle. The other two sides are called the legs or catheti (singular: cathetus) of the triangle. Right triangles obey the Pythagorean theorem: the sum of the squares of the lengths of the two legs is equal to the square of the length of the hypotenuse: $a^{2}+b^{2}=c^{2}$, where a and b are the lengths of the legs and c is the length of the hypotenuse. Special right triangles are right triangles with additional properties that make calculations involving them easier. One of the two most famous is the 3–4–5 right triangle, where $3^{2}+4^{2}=5^{2}$. The 3–4–5 triangle is also known as the Egyptian triangle. In this situation, 3, 4, and 5 are a Pythagorean triple. The other one is an isosceles triangle that has 2 angles measuring 45 degrees (45–45–90 triangle).
  • Triangles that do not have an angle measuring 90° are called oblique triangles.
• A triangle with all interior angles measuring less than 90° is an acute triangle or acute-angled triangle. If c is the length of the longest side, then $a^{2}+b^{2}>c^{2}$, where a and b are the lengths of the other sides.
• A triangle with one interior angle measuring more than 90° is an obtuse triangle or obtuse-angled triangle. If c is the length of the longest side, then $a^{2}+b^{2}<c^{2}$, where a and b are the lengths of the other sides.
• A triangle with an interior angle of 180° (and collinear vertices) is degenerate. A right degenerate triangle has collinear vertices, two of which are coincident.

A triangle that has two angles with the same measure also has two sides with the same length, and therefore it is an isosceles triangle. It follows that in a triangle where all angles have the same measure, all three sides have the same length, and therefore is equilateral.

• 

  Right

• 

  Obtuse

• 

  Acute