Difference between revisions of "Monotone Functions"
(Created page with "right|thumb|Figure 1. A monotonically non-decreasing function. Image:Monotonicity example2.svg|right|thumb|Figure 2. A monotonically non-...") |
|||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 2: | Line 2: | ||
[[Image:Monotonicity example2.svg|right|thumb|Figure 2. A monotonically non-increasing function]] | [[Image:Monotonicity example2.svg|right|thumb|Figure 2. A monotonically non-increasing function]] | ||
[[Image:Monotonicity example3.svg|right|thumb|Figure 3. A function that is ''not'' monotonic]] | [[Image:Monotonicity example3.svg|right|thumb|Figure 3. A function that is ''not'' monotonic]] | ||
| − | In | + | In mathematics, a '''monotonic function''' (or '''monotone function''') is a function between ordered sets that preserves or reverses the given order. This concept first arose in calculus, and was later generalized to the more abstract setting of order theory. |
== In calculus and analysis == | == In calculus and analysis == | ||
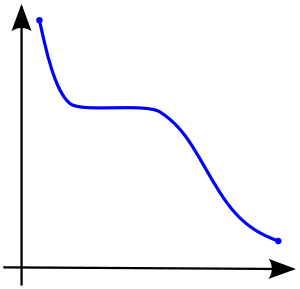
| − | In | + | In calculus, a function <math>f</math> defined on a subset of the real numbers with real values is called '''monotonic''' if and only if it is either entirely non-increasing, or entirely non-decreasing. That is, as per Fig. 1, a function that increases monotonically does not exclusively have to increase, it simply must not decrease. |
| − | A function is called '''monotonically increasing''' (also '''increasing''' or '''non-decreasing'''), | + | A function is called '''monotonically increasing''' (also '''increasing''' or '''non-decreasing'''), if for all <math>x</math> and <math>y</math> such that <math>x \leq y</math> one has <math>f\!\left(x\right) \leq f\!\left(y\right)</math>, so <math>f</math> preserves the order (see Figure 1). Likewise, a function is called '''monotonically decreasing''' (also '''decreasing''' or '''non-increasing''') if, whenever <math>x \leq y</math>, then <math>f\!\left(x\right) \geq f\!\left(y\right)</math>, so it ''reverses'' the order (see Figure 2). |
| − | If the order <math>\leq</math> in the definition of monotonicity is replaced by the strict order <math><</math>, then one obtains a stronger requirement. A function with this property is called '''strictly increasing''' (also '''increasing'''). | + | If the order <math>\leq</math> in the definition of monotonicity is replaced by the strict order <math><</math>, then one obtains a stronger requirement. A function with this property is called '''strictly increasing''' (also '''increasing'''). Again, by inverting the order symbol, one finds a corresponding concept called '''strictly decreasing''' (also '''decreasing'''). A function may be called '''strictly monotone''' if it is either strictly increasing or strictly decreasing. Functions that are strictly monotone are one-to-one (because for <math>x</math> not equal to <math>y</math>, either <math>x < y</math> or <math>x > y</math> and so, by monotonicity, either <math>f\!\left(x\right) < f\!\left(y\right)</math> or <math>f\!\left(x\right) > f\!\left(y\right)</math>, thus <math>f\!\left(x\right) \neq f\!\left(y\right)</math>.) |
If it is not clear that "increasing" and "decreasing" are taken to include the possibility of repeating the same value at successive arguments, one may use the terms '''weakly monotone''', '''weakly increasing''' and '''weakly decreasing''' to stress this possibility. | If it is not clear that "increasing" and "decreasing" are taken to include the possibility of repeating the same value at successive arguments, one may use the terms '''weakly monotone''', '''weakly increasing''' and '''weakly decreasing''' to stress this possibility. | ||
| Line 15: | Line 15: | ||
The terms "non-decreasing" and "non-increasing" should not be confused with the (much weaker) negative qualifications "not decreasing" and "not increasing". For example, the function of figure 3 first falls, then rises, then falls again. It is therefore not decreasing and not increasing, but it is neither non-decreasing nor non-increasing. | The terms "non-decreasing" and "non-increasing" should not be confused with the (much weaker) negative qualifications "not decreasing" and "not increasing". For example, the function of figure 3 first falls, then rises, then falls again. It is therefore not decreasing and not increasing, but it is neither non-decreasing nor non-increasing. | ||
| − | A function <math>f\!\left(x\right)</math> is said to be '''absolutely monotonic''' over an interval <math>\left(a, b\right)</math> if the derivatives of all orders of <math>f</math> are | + | A function <math>f\!\left(x\right)</math> is said to be '''absolutely monotonic''' over an interval <math>\left(a, b\right)</math> if the derivatives of all orders of <math>f</math> are nonnegative or all nonpositive at all points on the interval. |
=== Inverse of function === | === Inverse of function === | ||
| Line 23: | Line 23: | ||
However, a function ''y'' = ''g''(''x'') that is strictly monotonic, has an inverse function such that ''x'' = ''h''(''y'') because there is guaranteed to always be a one-to-one mapping from range to domain of the function. Also, a function can be said to be strictly monotonic on a range of values, and thus have an inverse on that range of value. For example, if ''y'' = ''g''(''x'') is strictly monotonic on the range [''a'',''b''], then it has an inverse ''x'' = ''h''(''y'') on the range [''g''(''a''), ''g''(''b'')], but we cannot say the entire range of the function has an inverse. | However, a function ''y'' = ''g''(''x'') that is strictly monotonic, has an inverse function such that ''x'' = ''h''(''y'') because there is guaranteed to always be a one-to-one mapping from range to domain of the function. Also, a function can be said to be strictly monotonic on a range of values, and thus have an inverse on that range of value. For example, if ''y'' = ''g''(''x'') is strictly monotonic on the range [''a'',''b''], then it has an inverse ''x'' = ''h''(''y'') on the range [''g''(''a''), ''g''(''b'')], but we cannot say the entire range of the function has an inverse. | ||
| − | Note, some textbooks | + | Note, some textbooks mistakenly state that an inverse exists for a monotonic function, when they really mean that an inverse exists for a strictly monotonic function. |
=== Monotonic transformation === | === Monotonic transformation === | ||
| − | The term '''monotonic transformation''' (or '''monotone transformation''') can also possibly cause some confusion because it refers to a transformation by a strictly increasing function. This is the case in economics with respect to the ordinal properties of a | + | The term '''monotonic transformation''' (or '''monotone transformation''') can also possibly cause some confusion because it refers to a transformation by a strictly increasing function. This is the case in economics with respect to the ordinal properties of a utility function being preserved across a monotonic transform (see also monotone preferences). In this context, what we are calling a "monotonic transformation" is, more accurately, called a "positive monotonic transformation", in order to distinguish it from a “negative monotonic transformation,” which reverses the order of the numbers. |
=== Some basic applications and results === | === Some basic applications and results === | ||
The following properties are true for a monotonic function <math>f\colon \mathbb{R} \to \mathbb{R}</math>: | The following properties are true for a monotonic function <math>f\colon \mathbb{R} \to \mathbb{R}</math>: | ||
| − | *<math>f</math> has | + | *<math>f</math> has limits from the right and from the left at every point of its domain; |
*<math>f</math> has a limit at positive or negative infinity (<math>\pm\infty</math>) of either a real number, <math>\infty</math>, or <math>-\infty</math>. | *<math>f</math> has a limit at positive or negative infinity (<math>\pm\infty</math>) of either a real number, <math>\infty</math>, or <math>-\infty</math>. | ||
| − | *<math>f</math> can only have | + | *<math>f</math> can only have jump discontinuities; |
| − | *<math>f</math> can only have | + | *<math>f</math> can only have countably many discontinuities in its domain. The discontinuities, however, do not necessarily consist of isolated points and may even be dense in an interval (''a'', ''b'').}} |
| − | These properties are the reason why monotonic functions are useful in technical work in | + | These properties are the reason why monotonic functions are useful in technical work in analysis. Some more facts about these functions are: |
| − | *if <math>f</math> is a monotonic function defined on an | + | *if <math>f</math> is a monotonic function defined on an interval <math>I</math>, then <math>f</math> is differentiable almost everywhere on <math>I</math>; i.e. the set of numbers <math>x</math> in <math>I</math> such that <math>f</math> is not differentiable in <math>x</math> has Lebesgue measure zero. In addition, this result cannot be improved to countable: see Cantor function. |
*if this set is countable, then <math>f</math> is absolutely continuous. | *if this set is countable, then <math>f</math> is absolutely continuous. | ||
| − | *if <math>f</math> is a monotonic function defined on an interval <math>\left[a, b\right]</math>, then <math>f</math> is | + | *if <math>f</math> is a monotonic function defined on an interval <math>\left[a, b\right]</math>, then <math>f</math> is Riemann integrable. |
| − | An important application of monotonic functions is in | + | An important application of monotonic functions is in probability theory. If <math>X</math> is a random variable, its cumulative distribution function <math>F_X\!\left(x\right) = \text{Prob}\!\left(X \leq x\right)</math> is a monotonically increasing function. |
| − | A function is '' | + | A function is ''unimodal'' if it is monotonically increasing up to some point (the ''mode'') and then monotonically decreasing. |
| − | When <math>f</math> is a ''strictly monotonic'' function, then <math>f</math> is | + | When <math>f</math> is a ''strictly monotonic'' function, then <math>f</math> is injective on its domain, and if <math>T</math> is the range of <math>f</math>, then there is an inverse function on <math>T</math> for <math>f</math>. In contrast, each constant function is monotonic, but not injective, and hence cannot have an inverse. |
== In topology == | == In topology == | ||
| Line 53: | Line 53: | ||
== In functional analysis == | == In functional analysis == | ||
| − | In | + | In functional analysis on a topological vector space <math>X</math>, a (possibly non-linear) operator <math>T: X \rightarrow X^*</math> is said to be a '''monotone operator''' if |
:<math>(Tu - Tv, u - v) \geq 0 \quad \forall u,v \in X.</math> | :<math>(Tu - Tv, u - v) \geq 0 \quad \forall u,v \in X.</math> | ||
| − | + | Kachurovskii's theorem shows that convex functions on Banach spaces have monotonic operators as their derivatives. | |
A subset <math>G</math> of <math>X \times X^*</math> is said to be a '''monotone set''' if for every pair <math>[u_1, w_1]</math> and <math>[u_2, w_2]</math> in <math>G</math>, | A subset <math>G</math> of <math>X \times X^*</math> is said to be a '''monotone set''' if for every pair <math>[u_1, w_1]</math> and <math>[u_2, w_2]</math> in <math>G</math>, | ||
| Line 64: | Line 64: | ||
<math>G</math> is said to be '''maximal monotone''' if it is maximal among all monotone sets in the sense of set inclusion. The graph of a monotone operator <math>G(T)</math> is a monotone set. A monotone operator is said to be '''maximal monotone''' if its graph is a '''maximal monotone set'''. | <math>G</math> is said to be '''maximal monotone''' if it is maximal among all monotone sets in the sense of set inclusion. The graph of a monotone operator <math>G(T)</math> is a monotone set. A monotone operator is said to be '''maximal monotone''' if its graph is a '''maximal monotone set'''. | ||
| + | |||
| + | == Licensing == | ||
| + | Content obtained and/or adapted from: | ||
| + | * [https://en.wikipedia.org/wiki/Monotonic_function Monotonic function, Wikipedia] under a CC BY-SA license | ||
Latest revision as of 11:15, 6 November 2021
In mathematics, a monotonic function (or monotone function) is a function between ordered sets that preserves or reverses the given order. This concept first arose in calculus, and was later generalized to the more abstract setting of order theory.
Contents
In calculus and analysis
In calculus, a function Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} defined on a subset of the real numbers with real values is called monotonic if and only if it is either entirely non-increasing, or entirely non-decreasing. That is, as per Fig. 1, a function that increases monotonically does not exclusively have to increase, it simply must not decrease.
A function is called monotonically increasing (also increasing or non-decreasing), if for all and such that one has , so preserves the order (see Figure 1). Likewise, a function is called monotonically decreasing (also decreasing or non-increasing) if, whenever , then , so it reverses the order (see Figure 2).
If the order in the definition of monotonicity is replaced by the strict order , then one obtains a stronger requirement. A function with this property is called strictly increasing (also increasing). Again, by inverting the order symbol, one finds a corresponding concept called strictly decreasing (also decreasing). A function may be called strictly monotone if it is either strictly increasing or strictly decreasing. Functions that are strictly monotone are one-to-one (because for not equal to , either or and so, by monotonicity, either or , thus Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f\!\left(x\right) \neq f\!\left(y\right)} .)
If it is not clear that "increasing" and "decreasing" are taken to include the possibility of repeating the same value at successive arguments, one may use the terms weakly monotone, weakly increasing and weakly decreasing to stress this possibility.
The terms "non-decreasing" and "non-increasing" should not be confused with the (much weaker) negative qualifications "not decreasing" and "not increasing". For example, the function of figure 3 first falls, then rises, then falls again. It is therefore not decreasing and not increasing, but it is neither non-decreasing nor non-increasing.
A function Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f\!\left(x\right)} is said to be absolutely monotonic over an interval if the derivatives of all orders of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} are nonnegative or all nonpositive at all points on the interval.
Inverse of function
A function that is monotonic, but not strictly monotonic, and thus constant on an interval, doesn't have an inverse. This is because in order for a function to have an inverse, there needs to be a one-to-one mapping from the range to the domain of the function. Since a monotonic function has some values that are constant in its domain, this means that there would be more than one value in the range that maps to this constant value.
However, a function y = g(x) that is strictly monotonic, has an inverse function such that x = h(y) because there is guaranteed to always be a one-to-one mapping from range to domain of the function. Also, a function can be said to be strictly monotonic on a range of values, and thus have an inverse on that range of value. For example, if y = g(x) is strictly monotonic on the range [a,b], then it has an inverse x = h(y) on the range [g(a), g(b)], but we cannot say the entire range of the function has an inverse.
Note, some textbooks mistakenly state that an inverse exists for a monotonic function, when they really mean that an inverse exists for a strictly monotonic function.
Monotonic transformation
The term monotonic transformation (or monotone transformation) can also possibly cause some confusion because it refers to a transformation by a strictly increasing function. This is the case in economics with respect to the ordinal properties of a utility function being preserved across a monotonic transform (see also monotone preferences). In this context, what we are calling a "monotonic transformation" is, more accurately, called a "positive monotonic transformation", in order to distinguish it from a “negative monotonic transformation,” which reverses the order of the numbers.
Some basic applications and results
The following properties are true for a monotonic function Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f\colon \mathbb{R} \to \mathbb{R}} :
- has limits from the right and from the left at every point of its domain;
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} has a limit at positive or negative infinity (Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \pm\infty} ) of either a real number, , or Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -\infty} .
- can only have jump discontinuities;
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} can only have countably many discontinuities in its domain. The discontinuities, however, do not necessarily consist of isolated points and may even be dense in an interval (a, b).}}
These properties are the reason why monotonic functions are useful in technical work in analysis. Some more facts about these functions are:
- if is a monotonic function defined on an interval Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle I} , then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} is differentiable almost everywhere on ; i.e. the set of numbers Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} in Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle I} such that is not differentiable in Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} has Lebesgue measure zero. In addition, this result cannot be improved to countable: see Cantor function.
- if this set is countable, then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} is absolutely continuous.
- if is a monotonic function defined on an interval Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left[a, b\right]} , then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} is Riemann integrable.
An important application of monotonic functions is in probability theory. If is a random variable, its cumulative distribution function Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_X\!\left(x\right) = \text{Prob}\!\left(X \leq x\right)} is a monotonically increasing function.
A function is unimodal if it is monotonically increasing up to some point (the mode) and then monotonically decreasing.
When Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} is a strictly monotonic function, then is injective on its domain, and if Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T} is the range of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} , then there is an inverse function on for Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} . In contrast, each constant function is monotonic, but not injective, and hence cannot have an inverse.
In topology
A map Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f: X \rightarrow Y} is said to be monotone if each of its fibers is connected; i.e. for each element in the (possibly empty) set is connected.
In functional analysis
In functional analysis on a topological vector space , a (possibly non-linear) operator is said to be a monotone operator if
Kachurovskii's theorem shows that convex functions on Banach spaces have monotonic operators as their derivatives.
A subset of is said to be a monotone set if for every pair and in ,
![{\displaystyle [u_{1},w_{1}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/42f2c67bc4887974d491ba4a419dc798ed50d8cd)
![{\displaystyle [u_{2},w_{2}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/32202d66739c2039a8b74e861330c713a44db704)
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (w_1 - w_2, u_1 - u_2) \geq 0.}
Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G} is said to be maximal monotone if it is maximal among all monotone sets in the sense of set inclusion. The graph of a monotone operator is a monotone set. A monotone operator is said to be maximal monotone if its graph is a maximal monotone set.
Licensing
Content obtained and/or adapted from:
- Monotonic function, Wikipedia under a CC BY-SA license
