Area of Polygons - Formulas

Area of Parallelogram

A parallelogram can be rearranged into a rectangle with the same area.

Animation for the area formula $K=bh$.

All of the area formulas for general convex quadrilaterals apply to parallelograms. Further formulas are specific to parallelograms:

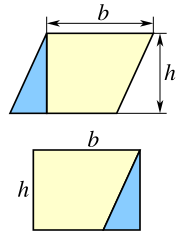
A parallelogram with base b and height h can be divided into a trapezoid and a right triangle, and rearranged into a rectangle, as shown in the figure to the left. This means that the area of a parallelogram is the same as that of a rectangle with the same base and height:

$K=bh.$

The area of the parallelogram is the area of the blue region, which is the interior of the parallelogram

The base × height area formula can also be derived using the figure to the right. The area K of the parallelogram to the right (the blue area) is the total area of the rectangle less the area of the two orange triangles. The area of the rectangle is

$K_{\text{rect}}=(B+A)\times H\,$

and the area of a single orange triangle is

$K_{\text{tri}}={\frac {A}{2}}\times H.\,$

Therefore, the area of the parallelogram is

$K=K_{\text{rect}}-2\times K_{\text{tri}}=((B+A)\times H)-(A\times H)=B\times H.$

Another area formula, for two sides B and C and angle θ, is

$K=B\cdot C\cdot \sin \theta .\,$

The area of a parallelogram with sides B and C (B ≠ C) and angle $\gamma$ at the intersection of the diagonals is given by

$K={\frac {|\tan \gamma |}{2}}\cdot \left|B^{2}-C^{2}\right|.$

When the parallelogram is specified from the lengths B and C of two adjacent sides together with the length D₁ of either diagonal, then the area can be found from Heron's formula. Specifically it is

$K=2{\sqrt {S(S-B)(S-C)(S-D_{1})}}$

where $S=(B+C+D_{1})/2$ and the leading factor 2 comes from the fact that the chosen diagonal divides the parallelogram into two congruent triangles.

Area of Triangle

Calculating the area T of a triangle is an elementary problem encountered often in many different situations. The best known and simplest formula is:

$T={\frac {1}{2}}bh$,

where b is the length of the base of the triangle, and h is the height or altitude of the triangle. The term "base" denotes any side, and "height" denotes the length of a perpendicular from the vertex opposite the base onto the line containing the base. In 499 CE Aryabhata, used this illustrated method in the Aryabhatiya (section 2.6).

Although simple, this formula is only useful if the height can be readily found, which is not always the case. For example, the surveyor of a triangular field might find it relatively easy to measure the length of each side, but relatively difficult to construct a 'height'. Various methods may be used in practice, depending on what is known about the triangle. The following is a selection of frequently used formulae for the area of a triangle.

Using trigonometry

Applying trigonometry to find the altitude h. The height of a triangle can be found through the application of trigonometry.

Knowing SAS: Using the labels in the image on the right, the altitude is $h=a\sin {\gamma }$. Substituting this in the formula $T={\frac {1}{2}}bh$ derived above, the area of the triangle can be expressed as:

$T={\frac {1}{2}}ab\sin {\gamma }={\frac {1}{2}}bc\sin {\alpha }={\frac {1}{2}}ca\sin {\beta }$

(where α is the interior angle at A, β is the interior angle at B, $\gamma$ is the interior angle at C and c is the line AB).

Furthermore, since sin α = sin (π − α) = sin (β + $\gamma$), and similarly for the other two angles:

$T={\frac {1}{2}}ab\sin(\alpha +\beta )={\frac {1}{2}}bc\sin(\beta +\gamma )={\frac {1}{2}}ca\sin(\gamma +\alpha )$.

Knowing AAS:

$T={\frac {b^{2}(\sin \alpha )(\sin(\alpha +\beta ))}{2\sin \beta }}$, and analogously if the known side is a or c.

Knowing ASA:

$T={\frac {a^{2}}{2(\cot \beta +\cot \gamma )}}={\frac {a^{2}(\sin \beta )(\sin \gamma )}{2\sin(\beta +\gamma )}}$, and analogously if the known side is b or c.

Using Heron's formula

The shape of the triangle is determined by the lengths of the sides. Therefore, the area can also be derived from the lengths of the sides. By Heron's formula:

$T={\sqrt {s(s-a)(s-b)(s-c)}}$ where $s={\tfrac {a+b+c}{2}}$ is the semiperimeter, or half of the triangle's perimeter.

Three other equivalent ways of writing Heron's formula are

$T={\frac {1}{4}}{\sqrt {(a^{2}+b^{2}+c^{2})^{2}-2(a^{4}+b^{4}+c^{4})}}$

$T={\frac {1}{4}}{\sqrt {2(a^{2}b^{2}+a^{2}c^{2}+b^{2}c^{2})-(a^{4}+b^{4}+c^{4})}}$

$T={\frac {1}{4}}{\sqrt {(a+b-c)(a-b+c)(-a+b+c)(a+b+c)}}$.

Area of Trapezoid

The area K of a trapezoid is given by

$K={\frac {a+b}{2}}\cdot h=mh$

where a and b are the lengths of the parallel sides, h is the height (the perpendicular distance between these sides), and m is the arithmetic mean of the lengths of the two parallel sides. In 499 AD Aryabhata, a great mathematician-astronomer from the classical age of Indian mathematics and Indian astronomy, used this method in the Aryabhatiya (section 2.8). This yields as a special case the well-known formula for the area of a triangle, by considering a triangle as a degenerate trapezoid in which one of the parallel sides has shrunk to a point.

The 7th-century Indian mathematician Bhāskara I derived the following formula for the area of a trapezoid with consecutive sides a, c, b, d:

$K={\frac {1}{2}}(a+b){\sqrt {c^{2}-{\frac {1}{4}}\left((b-a)+{\frac {c^{2}-d^{2}}{b-a}}\right)^{2}}}$

where a and b are parallel and b > a. This formula can be factored into a more symmetric version

$K={\frac {a+b}{4|b-a|}}{\sqrt {(-a+b+c+d)(a-b+c+d)(a-b+c-d)(a-b-c+d)}}.$

When one of the parallel sides has shrunk to a point (say a = 0), this formula reduces to Heron's formula for the area of a triangle.

Another equivalent formula for the area, which more closely resembles Heron's formula, is

$K={\frac {a+b}{|b-a|}}{\sqrt {(s-b)(s-a)(s-b-c)(s-b-d)}},$

where $s={\tfrac {1}{2}}(a+b+c+d)$ is the semiperimeter of the trapezoid. (This formula is similar to Brahmagupta's formula, but it differs from it, in that a trapezoid might not be cyclic (inscribed in a circle). The formula is also a special case of Bretschneider's formula for a general quadrilateral).

From Bretschneider's formula, it follows that

$K={\sqrt {{\frac {(ab^{2}-a^{2}b-ad^{2}+bc^{2})(ab^{2}-a^{2}b-ac^{2}+bd^{2})}{(2(b-a))^{2}}}-\left({\frac {b^{2}+d^{2}-a^{2}-c^{2}}{4}}\right)^{2}}}.$

The line that joins the midpoints of the parallel sides, bisects the area.

Licensing

Content obtained and/or adapted from:

• Parallelogram, Wikipedia under a CC BY-SA license
• Triangle, Wikipedia under a CC BY-SA license
• Trapezoid, Wikipedia under a CC BY-SA license