Difference between revisions of "Quadratic Equations"
| (9 intermediate revisions by the same user not shown) | |||
| Line 21: | Line 21: | ||
It may be possible to express a quadratic equation ''ax''<sup>2</sup> + ''bx'' + ''c'' = 0 as a product (''px'' + ''q'')(''rx'' + ''s'') = 0. In some cases, it is possible, by simple inspection, to determine values of ''p'', ''q'', ''r,'' and ''s'' that make the two forms equivalent to one another. If the quadratic equation is written in the second form, then the "Zero Factor Property" states that the quadratic equation is satisfied if ''px'' + ''q'' = 0 or ''rx'' + ''s'' = 0. Solving these two linear equations provides the roots of the quadratic. | It may be possible to express a quadratic equation ''ax''<sup>2</sup> + ''bx'' + ''c'' = 0 as a product (''px'' + ''q'')(''rx'' + ''s'') = 0. In some cases, it is possible, by simple inspection, to determine values of ''p'', ''q'', ''r,'' and ''s'' that make the two forms equivalent to one another. If the quadratic equation is written in the second form, then the "Zero Factor Property" states that the quadratic equation is satisfied if ''px'' + ''q'' = 0 or ''rx'' + ''s'' = 0. Solving these two linear equations provides the roots of the quadratic. | ||
| − | For most students, factoring by inspection is the first method of solving quadratic equations to which they are exposed. If one is given a quadratic equation in the form | + | For most students, factoring by inspection is the first method of solving quadratic equations to which they are exposed. If one is given a quadratic equation in the form ''x''<sup>2</sup> + ''bx'' + ''c'' = 0, the sought factorization has the form {{math|(''x'' + ''q'')(''x'' + ''s'')}}, and one has to find two numbers {{math|''q''}} and {{math|''s''}} that add up to {{math| ''b''}} and whose product is {{math|''c''}} (this is sometimes called "Vieta's rule". and is related to Vieta's formulas). As an example, {{math|''x''<sup>2</sup> + 5''x'' + 6}} factors as {{math|(''x'' + 3)(''x'' + 2)}}. The more general case where {{math|''a''}} does not equal {{math|1}} can require a considerable effort in trial and error guess-and-check, assuming that it can be factored at all by inspection. |
| − | Except for special cases such as where | + | Except for special cases such as where ''b'' = 0 or ''c'' = 0, factoring by inspection only works for quadratic equations that have rational roots. This means that the great majority of quadratic equations that arise in practical applications cannot be solved by factoring by inspection. |
===Completing the square=== | ===Completing the square=== | ||
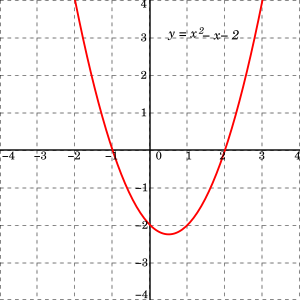
| − | [[File:Polynomialdeg2.svg|thumb|right|300px|Figure 2. For the | + | [[File:Polynomialdeg2.svg|thumb|right|300px|Figure 2. For the quadratic function ''y'' = ''x''<sup>2</sup> − ''x'' − 2, the points where the graph crosses the {{math|''x''}}-axis, ''x'' = −1 and ''x'' = 2, are the solutions of the quadratic equation ''x''<sup>2</sup> − ''x'' − 2 = 0. |
|alt=Figure 2 illustrates an x y plot of the quadratic function f of x equals x squared minus x minus 2. The x-coordinate of the points where the graph intersects the x-axis, x equals −1 and x equals 2, are the solutions of the quadratic equation x squared minus x minus 2 equals zero.]] | |alt=Figure 2 illustrates an x y plot of the quadratic function f of x equals x squared minus x minus 2. The x-coordinate of the points where the graph intersects the x-axis, x equals −1 and x equals 2, are the solutions of the quadratic equation x squared minus x minus 2 equals zero.]] | ||
The process of completing the square makes use of the algebraic identity | The process of completing the square makes use of the algebraic identity | ||
:<math>x^2+2hx+h^2 = (x+h)^2,</math> | :<math>x^2+2hx+h^2 = (x+h)^2,</math> | ||
| − | which represents a well-defined | + | which represents a well-defined algorithm that can be used to solve any quadratic equation. Starting with a quadratic equation in standard form, ''ax''<sup>2</sup> + ''bx'' + ''c'' = 0 |
#Divide each side by {{math|''a''}}, the coefficient of the squared term. | #Divide each side by {{math|''a''}}, the coefficient of the squared term. | ||
#Subtract the constant term {{math|''c''/''a''}} from both sides. | #Subtract the constant term {{math|''c''/''a''}} from both sides. | ||
| Line 38: | Line 38: | ||
#Solve each of the two linear equations. | #Solve each of the two linear equations. | ||
| − | We illustrate use of this algorithm by solving | + | We illustrate use of this algorithm by solving 2''x''<sup>2</sup> + 4''x'' − 4 = 0 |
:<math>1) \ x^2+2x-2=0</math> | :<math>1) \ x^2+2x-2=0</math> | ||
:<math>2) \ x^2+2x=2</math> | :<math>2) \ x^2+2x=2</math> | ||
| Line 46: | Line 46: | ||
:<math>6) \ x=-1\pm\sqrt{3}</math> | :<math>6) \ x=-1\pm\sqrt{3}</math> | ||
| − | The | + | The plus–minus symbol "±" indicates that both <math> x = -1 + \sqrt{3}</math> and <math> x = -1 - \sqrt{3}</math> are solutions of the quadratic equation. |
=== Quadratic formula and its derivation === | === Quadratic formula and its derivation === | ||
| − | + | Completing the square can be used to derive a general formula for solving quadratic equations, called the quadratic formula. The mathematical proof will now be briefly summarized. It can easily be seen, by polynomial expansion, that the following equation is equivalent to the quadratic equation: | |
:<math>\left(x+\frac{b}{2a}\right)^2=\frac{b^2-4ac}{4a^2}.</math> | :<math>\left(x+\frac{b}{2a}\right)^2=\frac{b^2-4ac}{4a^2}.</math> | ||
| − | Taking the | + | Taking the square root of both sides, and isolating {{math|''x''}}, gives: |
:<math>x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}.</math> | :<math>x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}.</math> | ||
| − | Some sources, particularly older ones, use alternative parameterizations of the quadratic equation such as | + | Some sources, particularly older ones, use alternative parameterizations of the quadratic equation such as ''ax''<sup>2</sup> + 2''bx'' + ''c'' = ''0'' or ''ax''<sup>2</sup> − 2''bx'' + ''c'' = 0 , where {{math|''b''}} has a magnitude one half of the more common one, possibly with opposite sign. These result in slightly different forms for the solution, but are otherwise equivalent. |
| − | A number of | + | A number of alternative derivations can be found in the literature. These proofs are simpler than the standard completing the square method, represent interesting applications of other frequently used techniques in algebra, or offer insight into other areas of mathematics. |
| − | A lesser known quadratic formula, as used in | + | A lesser known quadratic formula, as used in Muller's method provides the same roots via the equation |
:<math>x = \frac{2c}{-b \pm \sqrt {b^2-4ac}}.</math> | :<math>x = \frac{2c}{-b \pm \sqrt {b^2-4ac}}.</math> | ||
| − | This can be deduced from the standard quadratic formula by | + | This can be deduced from the standard quadratic formula by Vieta's formulas, which assert that the product of the roots is {{math|''c''/''a''}}. |
| − | One property of this form is that it yields one valid root when | + | One property of this form is that it yields one valid root when ''a'' = 0, while the other root contains division by zero, because when ''a'' = 0, the quadratic equation becomes a linear equation, which has one root. By contrast, in this case, the more common formula has a division by zero for one root and an indeterminate form {{math|0/0}} for the other root. On the other hand, when ''c'' = 0, the more common formula yields two correct roots whereas this form yields the zero root and an indeterminate form {{math|0/0}}. |
===Reduced quadratic equation=== | ===Reduced quadratic equation=== | ||
| − | It is sometimes convenient to reduce a quadratic equation so that its | + | It is sometimes convenient to reduce a quadratic equation so that its leading coefficient is one. This is done by dividing both sides by {{math|''a''}}, which is always possible since {{math|''a''}} is non-zero. This produces the ''reduced quadratic equation'': |
:<math>x^2+px+q=0,</math> | :<math>x^2+px+q=0,</math> | ||
| − | where | + | where ''p'' = ''b''/''a'' and ''q'' = ''c''/''a''. This monic polynomial equation has the same solutions as the original. |
The quadratic formula for the solutions of the reduced quadratic equation, written in terms of its coefficients, is: | The quadratic formula for the solutions of the reduced quadratic equation, written in terms of its coefficients, is: | ||
| Line 78: | Line 78: | ||
===Discriminant=== | ===Discriminant=== | ||
[[File:Quadratic eq discriminant.svg|thumb|right|Figure 3. Discriminant signs|alt=Figure 3. This figure plots three quadratic functions on a single Cartesian plane graph to illustrate the effects of discriminant values. When the discriminant, delta, is positive, the parabola intersects the {{math|''x''}}-axis at two points. When delta is zero, the vertex of the parabola touches the {{math|''x''}}-axis at a single point. When delta is negative, the parabola does not intersect the {{math|''x''}}-axis at all.]] | [[File:Quadratic eq discriminant.svg|thumb|right|Figure 3. Discriminant signs|alt=Figure 3. This figure plots three quadratic functions on a single Cartesian plane graph to illustrate the effects of discriminant values. When the discriminant, delta, is positive, the parabola intersects the {{math|''x''}}-axis at two points. When delta is zero, the vertex of the parabola touches the {{math|''x''}}-axis at a single point. When delta is negative, the parabola does not intersect the {{math|''x''}}-axis at all.]] | ||
| − | In the quadratic formula, the expression underneath the square root sign is called the '' | + | In the quadratic formula, the expression underneath the square root sign is called the ''discriminant'' of the quadratic equation, and is often represented using an upper case {{math|''D''}} or an upper case Greek delta: |
:<math>\Delta = b^2 - 4ac.</math> | :<math>\Delta = b^2 - 4ac.</math> | ||
A quadratic equation with ''real'' coefficients can have either one or two distinct real roots, or two distinct complex roots. In this case the discriminant determines the number and nature of the roots. There are three cases: | A quadratic equation with ''real'' coefficients can have either one or two distinct real roots, or two distinct complex roots. In this case the discriminant determines the number and nature of the roots. There are three cases: | ||
| Line 84: | Line 84: | ||
*If the discriminant is positive, then there are two distinct roots | *If the discriminant is positive, then there are two distinct roots | ||
::<math>\frac{-b + \sqrt {\Delta}}{2a} \quad\text{and}\quad \frac{-b - \sqrt {\Delta}}{2a},</math> | ::<math>\frac{-b + \sqrt {\Delta}}{2a} \quad\text{and}\quad \frac{-b - \sqrt {\Delta}}{2a},</math> | ||
| − | :both of which are real numbers. For quadratic equations with | + | :both of which are real numbers. For quadratic equations with rational coefficients, if the discriminant is a square number, then the roots are rational—in other cases they may be quadratic irrationals. |
| − | *If the discriminant is zero, then there is exactly one | + | *If the discriminant is zero, then there is exactly one real root |
::<math>-\frac{b}{2a},</math> | ::<math>-\frac{b}{2a},</math> | ||
| − | :sometimes called a repeated or | + | :sometimes called a repeated or double root. |
| − | *If the discriminant is negative, then there are no real roots. Rather, there are two distinct (non-real) | + | *If the discriminant is negative, then there are no real roots. Rather, there are two distinct (non-real) complex roots |
::<math> -\frac{b}{2a} + i \frac{\sqrt {-\Delta}}{2a} \quad\text{and}\quad -\frac{b}{2a} - i \frac{\sqrt {-\Delta}}{2a},</math> | ::<math> -\frac{b}{2a} + i \frac{\sqrt {-\Delta}}{2a} \quad\text{and}\quad -\frac{b}{2a} - i \frac{\sqrt {-\Delta}}{2a},</math> | ||
| − | :which are | + | :which are complex conjugates of each other. In these expressions {{math|''i''}} is the imaginary unit. |
Thus the roots are distinct if and only if the discriminant is non-zero, and the roots are real if and only if the discriminant is non-negative. | Thus the roots are distinct if and only if the discriminant is non-zero, and the roots are real if and only if the discriminant is non-negative. | ||
===Geometric interpretation=== | ===Geometric interpretation=== | ||
| + | [[File:Quadratic function graph key values.svg|thumb|Quadratic function graph key values|Graph of <math> y = ax^2 + bx + c</math>, where ''a'' and the discriminant <math> b^2 - 4ac</math> are positive, with <li> Roots and ''y''-intercept in <span style="color:red"> red</span></li> <li> Vertex and axis of symmetry in <span style="color:blue"> blue </span></li> <li>Focus and directrix in <span style="color:#FF1694">pink</span></li>]] | ||
| + | The function ''f''(''x'') = ''ax''<sup>2</sup> + ''bx'' + ''c'' is a quadratic function. The graph of any quadratic function has the same general shape, which is called a parabola. The location and size of the parabola, and how it opens, depend on the values of {{math|''a''}}, {{math|''b''}}, and {{math|''c''}}. As shown in Figure 1, if {{math|''a'' > 0}}, the parabola has a minimum point and opens upward. If {{math|''a'' < 0}}, the parabola has a maximum point and opens downward. The extreme point of the parabola, whether minimum or maximum, corresponds to its vertex. The ''{{math|x}}-coordinate'' of the vertex will be located at <math>\scriptstyle x=\tfrac{-b}{2a}</math>, and the ''{{math|y}}-coordinate'' of the vertex may be found by substituting this ''{{math|x}}-value'' into the function. The ''{{math|y}}-intercept'' is located at the point {{math|(0, ''c'')}}. | ||
| − | + | The solutions of the quadratic equation ''ax''<sup>2</sup> + ''bx'' + ''c'' = 0 correspond to the roots of the function ''f''(''x'') = ''ax''<sup>2</sup> + ''bx'' + ''c'', since they are the values of {{math|''x''}} for which ''f''(''x'') = 0. As shown in Figure 2, if {{math|''a''}}, {{math|''b''}}, and {{math|''c''}} are real numbers and the domain of {{math|''f''}} is the set of real numbers, then the roots of {{math|''f''}} are exactly the {{math|''x''}}-coordinates of the points where the graph touches the {{math|''x''}}-axis. As shown in Figure 3, if the discriminant is positive, the graph touches the {{math|''x''}}-axis at two points; if zero, the graph touches at one point; and if negative, the graph does not touch the {{math|''x''}}-axis. | |
| − | |||
| − | The solutions of the quadratic equation | ||
===Quadratic factorization=== | ===Quadratic factorization=== | ||
| Line 107: | Line 107: | ||
is a factor of the polynomial | is a factor of the polynomial | ||
: <math>ax^2+bx+c</math> | : <math>ax^2+bx+c</math> | ||
| − | if and only if {{math|''r''}} is a | + | if and only if {{math|''r''}} is a root of the quadratic equation |
: <math>ax^2+bx+c=0.</math> | : <math>ax^2+bx+c=0.</math> | ||
It follows from the quadratic formula that | It follows from the quadratic formula that | ||
: <math>ax^2+bx+c = a \left( x - \frac{-b + \sqrt {b^2-4ac}}{2a} \right) \left( x - \frac{-b - \sqrt {b^2-4ac}}{2a} \right).</math> | : <math>ax^2+bx+c = a \left( x - \frac{-b + \sqrt {b^2-4ac}}{2a} \right) \left( x - \frac{-b - \sqrt {b^2-4ac}}{2a} \right).</math> | ||
| − | In the special case | + | In the special case ''b''<sup>2</sup> = 4''ac'' where the quadratic has only one distinct root (''i.e.'' the discriminant is zero), the quadratic polynomial can be factored as |
:<math>ax^2+bx+c = a \left( x + \frac{b}{2a} \right)^2.</math> | :<math>ax^2+bx+c = a \left( x + \frac{b}{2a} \right)^2.</math> | ||
===Graphical solution=== | ===Graphical solution=== | ||
| − | + | ||
| − | [[Image:Visual.complex.root.finding.png|240px|right|thumb|A quadratic function without real root: | + | [[Image:Visual.complex.root.finding.png|240px|right|thumb|A quadratic function without real root: ''y'' = (''x'' − 5)<sup>2</sup> + 9. The "3" is the imaginary part of the ''x''-intercept. The real part is the ''x''-coordinate of the vertex. Thus the roots are 5 ± 3''i''.]] |
The solutions of the quadratic equation | The solutions of the quadratic equation | ||
:<math>ax^2+bx+c=0</math> | :<math>ax^2+bx+c=0</math> | ||
| − | may be deduced from the | + | may be deduced from the graph of the quadratic function |
:<math>y=ax^2+bx+c,</math> | :<math>y=ax^2+bx+c,</math> | ||
| − | which is a | + | which is a parabola. |
| − | If the parabola intersects the {{mvar|x}}-axis in two points, there are two real | + | If the parabola intersects the {{mvar|x}}-axis in two points, there are two real roots, which are the {{mvar|x}}-coordinates of these two points (also called {{mvar|x}}-intercept). |
| − | If the parabola is | + | If the parabola is tangent to the {{mvar|x}}-axis, there is a double root, which is the {{mvar|x}}-coordinate of the contact point between the graph and parabola. |
| − | If the parabola does not intersect the {{mvar|x}}-axis, there are two | + | If the parabola does not intersect the {{mvar|x}}-axis, there are two complex conjugate roots. Although these roots cannot be visualized on the graph, their real and imaginary parts can be. |
Let {{mvar|h}} and {{mvar|k}} be respectively the {{mvar|x}}-coordinate and the {{mvar|y}}-coordinate of the vertex of the parabola (that is the point with maximal or minimal {{mvar|y}}-coordinate. The quadratic function may be rewritten | Let {{mvar|h}} and {{mvar|k}} be respectively the {{mvar|x}}-coordinate and the {{mvar|y}}-coordinate of the vertex of the parabola (that is the point with maximal or minimal {{mvar|y}}-coordinate. The quadratic function may be rewritten | ||
| Line 138: | Line 138: | ||
===Avoiding loss of significance=== | ===Avoiding loss of significance=== | ||
| − | Although the quadratic formula provides an exact solution, the result is not exact if | + | Although the quadratic formula provides an exact solution, the result is not exact if real numbers are approximated during the computation, as usual in numerical analysis, where real numbers are approximated by floating point numbers (called "reals" in many programming languages). In this context, the quadratic formula is not completely stable. |
| − | This occurs when the roots have different | + | This occurs when the roots have different order of magnitude, or, equivalently, when {{math|''b''<sup>2</sup>}} and {{math|''b''<sup>2</sup> − 4''ac''}} are close in magnitude. In this case, the subtraction of two nearly equal numbers will cause loss of significance or catastrophic cancellation in the smaller root. To avoid this, the root that is smaller in magnitude, {{math|''r''}}, can be computed as <math>(c/a)/R</math> where {{math|''R''}} is the root that is bigger in magnitude. |
| − | A second form of cancellation can occur between the terms {{math|''b''<sup>2</sup>}} and {{math|4''ac''}} of the discriminant, that is when the two roots are very close. This can lead to loss of up to half of correct significant figures in the roots. | + | A second form of cancellation can occur between the terms {{math|''b''<sup>2</sup>}} and {{math|4''ac''}} of the discriminant, that is when the two roots are very close. This can lead to loss of up to half of correct significant figures in the roots. |
==Examples and applications== | ==Examples and applications== | ||
| − | [[File:La Jolla Cove cliff diving - 02.jpg|thumb|The trajectory of the cliff jumper is | + | [[File:La Jolla Cove cliff diving - 02.jpg|thumb|The trajectory of the cliff jumper is parabolic because horizontal displacement is a linear function of time <math>x=v_x t</math>, while vertical displacement is a quadratic function of time <math>y=\tfrac{1}{2} at^2+v_y t+h</math>. As a result, the path follows quadratic equation <math>y=\tfrac{a}{2v_x^2} x^2+\tfrac{v_y}{v_x} x+h</math>, where <math>v_x</math> and <math>v_y</math> are horizontal and vertical components of the original velocity, {{math|a}} is gravitational acceleration and {{math|h}} is original height. The {{math|a}} value should be considered negative here, as its direction (downwards) is opposite to the height measurement (upwards).]] |
| − | The | + | The golden ratio is found as the positive solution of the quadratic equation <math>x^2-x-1=0.</math> |
| − | |||
| − | |||
| − | + | The equations of the circle and the other conic sections—ellipses, parabolas, and hyperbolas—are quadratic equations in two variables. | |
| − | + | Given the cosine or sine of an angle, finding the cosine or sine of the angle that is half as large involves solving a quadratic equation. | |
| − | + | The process of simplifying expressions involving the square root of an expression involving the square root of another expression involves finding the two solutions of a quadratic equation. | |
| − | + | Descartes' theorem states that for every four kissing (mutually tangent) circles, their radii satisfy a particular quadratic equation. | |
| − | + | The equation given by Fuss' theorem, giving the relation among the radius of a bicentric quadrilateral's inscribed circle, the radius of its circumscribed circle, and the distance between the centers of those circles, can be expressed as a quadratic equation for which the distance between the two circles' centers in terms of their radii is one of the solutions. The other solution of the same equation in terms of the relevant radii gives the distance between the circumscribed circle's center and the center of the excircle of an ex-tangential quadrilateral. | |
| + | Critical points of a cubic function and inflection points of a quartic function are found by solving a quadratic equation. | ||
==Resources== | ==Resources== | ||
Latest revision as of 17:38, 22 January 2022
| The quadratic formula for the roots of the general quadratic equation |
In algebra, a quadratic equation (from the Latin quadratus for "square") is any equation that can be rearranged in standard form as
where x represents an unknown, and a, b, and c represent known numbers, where a ≠ 0. If a = 0, then the equation is linear, not quadratic, as there is no term. The numbers a, b, and c are the coefficients of the equation and may be distinguished by calling them, respectively, the quadratic coefficient, the linear coefficient and the constant or free term.
The values of x that satisfy the equation are called solutions of the equation, and roots or zeros of the expression on its left-hand side. A quadratic equation has at most two solutions. If there is only one solution, one says that it is a double root. If all the coefficients are real numbers, there are either two real solutions, or a single real double root, or two complex solutions. A quadratic equation always has two roots, if complex roots are included and a double root is counted for two. A quadratic equation can be factored into an equivalent equation
where r and s are the solutions for x. Completing the square on a quadratic equation in standard form results in the quadratic formula, which expresses the solutions in terms of a, b, and c. Solutions to problems that can be expressed in terms of quadratic equations were known as early as 2000 BC.
Because the quadratic equation involves only one unknown, it is called "univariate". The quadratic equation contains only powers of x that are non-negative integers, and therefore it is a polynomial equation. In particular, it is a second-degree polynomial equation, since the greatest power is two.
Solving the quadratic equation
A quadratic equation with real or complex coefficients has two solutions, called roots. These two solutions may or may not be distinct, and they may or may not be real.
Factoring by inspection
It may be possible to express a quadratic equation ax2 + bx + c = 0 as a product (px + q)(rx + s) = 0. In some cases, it is possible, by simple inspection, to determine values of p, q, r, and s that make the two forms equivalent to one another. If the quadratic equation is written in the second form, then the "Zero Factor Property" states that the quadratic equation is satisfied if px + q = 0 or rx + s = 0. Solving these two linear equations provides the roots of the quadratic.
For most students, factoring by inspection is the first method of solving quadratic equations to which they are exposed. If one is given a quadratic equation in the form x2 + bx + c = 0, the sought factorization has the form (x + q)(x + s), and one has to find two numbers q and s that add up to b and whose product is c (this is sometimes called "Vieta's rule". and is related to Vieta's formulas). As an example, x2 + 5x + 6 factors as (x + 3)(x + 2). The more general case where a does not equal 1 can require a considerable effort in trial and error guess-and-check, assuming that it can be factored at all by inspection.
Except for special cases such as where b = 0 or c = 0, factoring by inspection only works for quadratic equations that have rational roots. This means that the great majority of quadratic equations that arise in practical applications cannot be solved by factoring by inspection.
Completing the square
The process of completing the square makes use of the algebraic identity
which represents a well-defined algorithm that can be used to solve any quadratic equation. Starting with a quadratic equation in standard form, ax2 + bx + c = 0
- Divide each side by a, the coefficient of the squared term.
- Subtract the constant term c/a from both sides.
- Add the square of one-half of b/a, the coefficient of x, to both sides. This "completes the square", converting the left side into a perfect square.
- Write the left side as a square and simplify the right side if necessary.
- Produce two linear equations by equating the square root of the left side with the positive and negative square roots of the right side.
- Solve each of the two linear equations.
We illustrate use of this algorithm by solving 2x2 + 4x − 4 = 0
The plus–minus symbol "±" indicates that both and are solutions of the quadratic equation.
Quadratic formula and its derivation
Completing the square can be used to derive a general formula for solving quadratic equations, called the quadratic formula. The mathematical proof will now be briefly summarized. It can easily be seen, by polynomial expansion, that the following equation is equivalent to the quadratic equation:
Taking the square root of both sides, and isolating x, gives:
Some sources, particularly older ones, use alternative parameterizations of the quadratic equation such as ax2 + 2bx + c = 0 or ax2 − 2bx + c = 0 , where b has a magnitude one half of the more common one, possibly with opposite sign. These result in slightly different forms for the solution, but are otherwise equivalent.
A number of alternative derivations can be found in the literature. These proofs are simpler than the standard completing the square method, represent interesting applications of other frequently used techniques in algebra, or offer insight into other areas of mathematics.
A lesser known quadratic formula, as used in Muller's method provides the same roots via the equation
This can be deduced from the standard quadratic formula by Vieta's formulas, which assert that the product of the roots is c/a.
One property of this form is that it yields one valid root when a = 0, while the other root contains division by zero, because when a = 0, the quadratic equation becomes a linear equation, which has one root. By contrast, in this case, the more common formula has a division by zero for one root and an indeterminate form 0/0 for the other root. On the other hand, when c = 0, the more common formula yields two correct roots whereas this form yields the zero root and an indeterminate form 0/0.
Reduced quadratic equation
It is sometimes convenient to reduce a quadratic equation so that its leading coefficient is one. This is done by dividing both sides by a, which is always possible since a is non-zero. This produces the reduced quadratic equation:
where p = b/a and q = c/a. This monic polynomial equation has the same solutions as the original.
The quadratic formula for the solutions of the reduced quadratic equation, written in terms of its coefficients, is:
or equivalently:
Discriminant
In the quadratic formula, the expression underneath the square root sign is called the discriminant of the quadratic equation, and is often represented using an upper case D or an upper case Greek delta:
A quadratic equation with real coefficients can have either one or two distinct real roots, or two distinct complex roots. In this case the discriminant determines the number and nature of the roots. There are three cases:
- If the discriminant is positive, then there are two distinct roots
- both of which are real numbers. For quadratic equations with rational coefficients, if the discriminant is a square number, then the roots are rational—in other cases they may be quadratic irrationals.
- If the discriminant is zero, then there is exactly one real root
- sometimes called a repeated or double root.
- If the discriminant is negative, then there are no real roots. Rather, there are two distinct (non-real) complex roots
- which are complex conjugates of each other. In these expressions i is the imaginary unit.
Thus the roots are distinct if and only if the discriminant is non-zero, and the roots are real if and only if the discriminant is non-negative.
Geometric interpretation
The function f(x) = ax2 + bx + c is a quadratic function. The graph of any quadratic function has the same general shape, which is called a parabola. The location and size of the parabola, and how it opens, depend on the values of a, b, and c. As shown in Figure 1, if a > 0, the parabola has a minimum point and opens upward. If a < 0, the parabola has a maximum point and opens downward. The extreme point of the parabola, whether minimum or maximum, corresponds to its vertex. The x-coordinate of the vertex will be located at , and the y-coordinate of the vertex may be found by substituting this x-value into the function. The y-intercept is located at the point (0, c).
The solutions of the quadratic equation ax2 + bx + c = 0 correspond to the roots of the function f(x) = ax2 + bx + c, since they are the values of x for which f(x) = 0. As shown in Figure 2, if a, b, and c are real numbers and the domain of f is the set of real numbers, then the roots of f are exactly the x-coordinates of the points where the graph touches the x-axis. As shown in Figure 3, if the discriminant is positive, the graph touches the x-axis at two points; if zero, the graph touches at one point; and if negative, the graph does not touch the x-axis.
Quadratic factorization
The term
is a factor of the polynomial
if and only if r is a root of the quadratic equation
It follows from the quadratic formula that
In the special case b2 = 4ac where the quadratic has only one distinct root (i.e. the discriminant is zero), the quadratic polynomial can be factored as
Graphical solution
The solutions of the quadratic equation
may be deduced from the graph of the quadratic function
which is a parabola.
If the parabola intersects the x-axis in two points, there are two real roots, which are the x-coordinates of these two points (also called x-intercept).
If the parabola is tangent to the x-axis, there is a double root, which is the x-coordinate of the contact point between the graph and parabola.
If the parabola does not intersect the x-axis, there are two complex conjugate roots. Although these roots cannot be visualized on the graph, their real and imaginary parts can be.
Let h and k be respectively the x-coordinate and the y-coordinate of the vertex of the parabola (that is the point with maximal or minimal y-coordinate. The quadratic function may be rewritten
Let d be the distance between the point of y-coordinate 2k on the axis of the parabola, and a point on the parabola with the same y-coordinate (see the figure; there are two such points, which give the same distance, because of the symmetry of the parabola). Then the real part of the roots is h, and their imaginary part are ±d. That is, the roots are
or in the case of the example of the figure
Avoiding loss of significance
Although the quadratic formula provides an exact solution, the result is not exact if real numbers are approximated during the computation, as usual in numerical analysis, where real numbers are approximated by floating point numbers (called "reals" in many programming languages). In this context, the quadratic formula is not completely stable.
This occurs when the roots have different order of magnitude, or, equivalently, when b2 and b2 − 4ac are close in magnitude. In this case, the subtraction of two nearly equal numbers will cause loss of significance or catastrophic cancellation in the smaller root. To avoid this, the root that is smaller in magnitude, r, can be computed as where R is the root that is bigger in magnitude.
A second form of cancellation can occur between the terms b2 and 4ac of the discriminant, that is when the two roots are very close. This can lead to loss of up to half of correct significant figures in the roots.
Examples and applications

The golden ratio is found as the positive solution of the quadratic equation
The equations of the circle and the other conic sections—ellipses, parabolas, and hyperbolas—are quadratic equations in two variables.
Given the cosine or sine of an angle, finding the cosine or sine of the angle that is half as large involves solving a quadratic equation.
The process of simplifying expressions involving the square root of an expression involving the square root of another expression involves finding the two solutions of a quadratic equation.
Descartes' theorem states that for every four kissing (mutually tangent) circles, their radii satisfy a particular quadratic equation.
The equation given by Fuss' theorem, giving the relation among the radius of a bicentric quadrilateral's inscribed circle, the radius of its circumscribed circle, and the distance between the centers of those circles, can be expressed as a quadratic equation for which the distance between the two circles' centers in terms of their radii is one of the solutions. The other solution of the same equation in terms of the relevant radii gives the distance between the circumscribed circle's center and the center of the excircle of an ex-tangential quadrilateral.
Critical points of a cubic function and inflection points of a quartic function are found by solving a quadratic equation.
Resources
- Quadratic Functions, Book Chapters
- Guided Notes
Licensing
Content obtained and/or adapted from:
- Quadratic equation, Wikipedia under a CC BY-SA license