Difference between revisions of "Absolute Value Functions"
Jump to navigation
Jump to search
| Line 1: | Line 1: | ||
==Introduction== | ==Introduction== | ||
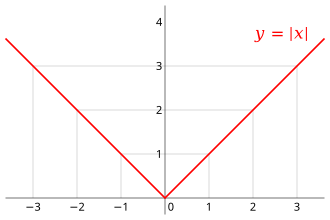
[[Image:Absolute value.svg|thumb|The graph of the absolute value function for real numbers]] | [[Image:Absolute value.svg|thumb|The graph of the absolute value function for real numbers]] | ||
| − | [[ | + | [[Image:Graph abs(x^2-4).png|thumb|The graph of the function <math> y = |5x + 5| </math>]] |
In mathematics, the absolute value or modulus of a real number x, denoted |x|, is the non-negative value of x without regard to its sign. Namely, |x| = x if x is positive, and |x| = −x if x is negative (in which case −x is positive), and |0| = 0. For example, the absolute value of 3 is 3, and the absolute value of −3 is also 3. The absolute value of a number may be thought of as its "distance from zero". The absolute value function f(x) = |a| can be expressed as a piecewise function, where f(x) = a when a ≥ 0, and f(x) = -a when a < 0. We can use this to help us visualize, graph, or solve other absolute value functions. | In mathematics, the absolute value or modulus of a real number x, denoted |x|, is the non-negative value of x without regard to its sign. Namely, |x| = x if x is positive, and |x| = −x if x is negative (in which case −x is positive), and |0| = 0. For example, the absolute value of 3 is 3, and the absolute value of −3 is also 3. The absolute value of a number may be thought of as its "distance from zero". The absolute value function f(x) = |a| can be expressed as a piecewise function, where f(x) = a when a ≥ 0, and f(x) = -a when a < 0. We can use this to help us visualize, graph, or solve other absolute value functions. | ||
| Line 7: | Line 7: | ||
* <math> g(x) = |5x + 5| </math>. 5x + 5 ≥ 0 when x ≥ -1, and 5x + 5 < 0 when x < -1. So, g(x) = 5x + 5 when x ≥ -1, and g(x) = -5x - 5 when x < -1. | * <math> g(x) = |5x + 5| </math>. 5x + 5 ≥ 0 when x ≥ -1, and 5x + 5 < 0 when x < -1. So, g(x) = 5x + 5 when x ≥ -1, and g(x) = -5x - 5 when x < -1. | ||
* <math> h(x) = |x^2 - 4| </math>. <math> x^2 - 4 \ge 0 </math> when -2 ≥ x or x ≥ 2. So, <math> h(x) = x^2 - 4 </math> when -2 ≥ x or x ≥ 2, and <math> h(x) = -x^2 + 4 </math> when -2 < x < 2. | * <math> h(x) = |x^2 - 4| </math>. <math> x^2 - 4 \ge 0 </math> when -2 ≥ x or x ≥ 2. So, <math> h(x) = x^2 - 4 </math> when -2 ≥ x or x ≥ 2, and <math> h(x) = -x^2 + 4 </math> when -2 < x < 2. | ||
| − | |||
| − | |||
==Resources== | ==Resources== | ||
Revision as of 12:12, 15 September 2021
Introduction
File:Absolute value.svg
The graph of the absolute value function for real numbers
.png)
In mathematics, the absolute value or modulus of a real number x, denoted |x|, is the non-negative value of x without regard to its sign. Namely, |x| = x if x is positive, and |x| = −x if x is negative (in which case −x is positive), and |0| = 0. For example, the absolute value of 3 is 3, and the absolute value of −3 is also 3. The absolute value of a number may be thought of as its "distance from zero". The absolute value function f(x) = |a| can be expressed as a piecewise function, where f(x) = a when a ≥ 0, and f(x) = -a when a < 0. We can use this to help us visualize, graph, or solve other absolute value functions.
Here are some examples of absolute value functions:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle g(x) = |5x + 5| } . 5x + 5 ≥ 0 when x ≥ -1, and 5x + 5 < 0 when x < -1. So, g(x) = 5x + 5 when x ≥ -1, and g(x) = -5x - 5 when x < -1.
- . when -2 ≥ x or x ≥ 2. So, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h(x) = x^2 - 4 } when -2 ≥ x or x ≥ 2, and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h(x) = -x^2 + 4 } when -2 < x < 2.
