Difference between revisions of "Graphs of Polynomials"
Jump to navigation
Jump to search
| Line 11: | Line 11: | ||
* The Tutte polynomial, a polynomial in two variables that can be defined (after a small change of variables) as the generating function of the numbers of connected components of induced subgraphs of the given graph, parameterized by the number of vertices in the subgraph. | * The Tutte polynomial, a polynomial in two variables that can be defined (after a small change of variables) as the generating function of the numbers of connected components of induced subgraphs of the given graph, parameterized by the number of vertices in the subgraph. | ||
| + | ===Graphs=== | ||
| + | <div class="floatright"> | ||
| + | <gallery perrow="2" widths="200px" heights="200px"> | ||
| + | File:Algebra1 fnz fig037 pc.svg|Polynomial of degree 0:<br/><small>{{math|''f''(''x'')}} = 2</small> | ||
| + | File:Fonction de Sophie Germain.png|Polynomial of degree 1:<br/><small>{{math|''f''(''x'')}} = 2''x'' + 1</small> | ||
| + | File:Polynomialdeg2.svg|Polynomial of degree 2:<br/><small>{{math|''f''(''x'')}} = ''x''<sup>2</sup> − ''x'' − 2<br/> = (''x'' + 1)(''x'' − 2)</small> | ||
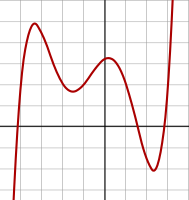
| + | File:Polynomialdeg3.svg|Polynomial of degree 3:<br/><small>{{math|''f''(''x'')}} = ''x''<sup>3</sup>/4 + 3''x''<sup>2</sup>/4 − 3''x''/2 − 2<br/> = 1/4 (''x'' + 4)(''x'' + 1)(''x'' − 2)</small> | ||
| + | File:Polynomialdeg4.svg|Polynomial of degree 4:<br/><small>{{math|''f''(''x'')}} = 1/14 (''x'' + 4)(''x'' + 1)(''x'' − 1)(''x'' − 3) <br/>+ 0.5</small> | ||
| + | File:Quintic polynomial.svg|Polynomial of degree 5:<br/><small>{{math|''f''(''x'')}} = 1/20 (''x'' + 4)(''x'' + 2)(''x'' + 1)(''x'' − 1)<br/>(''x'' − 3) + 2</small> | ||
| + | File:Sextic Graph.svg|Polynomial of degree 6:<br/><small>{{math|''f''(''x'')}} = 1/100 (''x''<sup>6</sup> − 2''x'' <sup>5</sup> − 26''x''<sup>4</sup> + 28''x''<sup>3</sup><br/>{{math|+ 145''x''<sup>2</sup> − 26''x'' − 80)}}</small> | ||
| + | File:Septic graph.svg|Polynomial of degree 7:<br/><small>{{math|''f''(''x'')}} = (''x'' − 3)(''x'' − 2)(''x'' − 1)(''x'')(''x'' + 1)(''x'' + 2)<br/>{{math|(''x'' + 3)}}</small> | ||
| + | </gallery> | ||
| + | </div> | ||
| + | A polynomial function in one real variable can be represented by a graph. | ||
| + | <ul> | ||
| + | <li> | ||
| + | The graph of the zero polynomial | ||
| + | :{{math|1=''f''(''x'') = 0}} | ||
| + | |||
| + | |||
| + | is the {{math|''x''}}-axis. | ||
| + | </li> | ||
| + | <li> | ||
| + | The graph of a degree 0 polynomial | ||
| + | :{{math|1=''f''(''x'') = ''a''<sub>0</sub>}}, where {{math|''a''<sub>0</sub> ≠ 0}}, | ||
| + | |||
| + | is a horizontal line with {{math|''y''}}-intercept {{math|''a''<sub>0</sub>}} | ||
| + | </li> | ||
| + | <li> | ||
| + | The graph of a degree 1 polynomial (or linear function) | ||
| + | :{{math|1=''f''(''x'') = ''a''<sub>0</sub> + ''a''<sub>1</sub>''x''}}, where {{math|''a''<sub>1</sub> ≠ 0}}, | ||
| + | |||
| + | |||
| + | is an oblique line with {{math|''y''}}-intercept {{math|''a''<sub>0</sub>}} and slope {{math|''a''<sub>1</sub>}}. | ||
| + | </li> | ||
| + | <li> | ||
| + | The graph of a degree 2 polynomial | ||
| + | :{{math|1=''f''(''x'') = ''a''<sub>0</sub> + ''a''<sub>1</sub>''x'' + ''a''<sub>2</sub>''x''<sup>2</sup>}}, where {{math|''a''<sub>2</sub> ≠ 0}} | ||
| + | |||
| + | is a parabola. | ||
| + | </li> | ||
| + | <li> | ||
| + | The graph of a degree 3 polynomial | ||
| + | :{{math|1=''f''(''x'') = ''a''<sub>0</sub> + ''a''<sub>1</sub>''x'' + ''a''<sub>2</sub>''x''<sup>2</sup> + ''a''<sub>3</sub>''x''<sup>3</sup>}}, where {{math|''a''<sub>3</sub> ≠ 0}} | ||
| + | |||
| + | is a cubic curve. | ||
| + | </li> | ||
| + | <li> | ||
| + | The graph of any polynomial with degree 2 or greater | ||
| + | :{{math|1=''f''(''x'') = ''a''<sub>0</sub> + ''a''<sub>1</sub>''x'' + ''a''<sub>2</sub>''x''<sup>2</sup> + ⋯ + ''a''<sub>''n''</sub>''x''<sup>''n''</sup>}}, where {{math|''a''<sub>''n''</sub> ≠ 0 and ''n'' ≥ 2}} | ||
| + | |||
| + | is a continuous non-linear curve. | ||
| + | </li> | ||
| + | </ul> | ||
| + | |||
| + | A non-constant polynomial function tends to infinity when the variable increases indefinitely (in absolute value). If the degree is higher than one, the graph does not have any asymptote. It has two parabolic branches with vertical direction (one branch for positive ''x'' and one for negative ''x''). | ||
| + | |||
| + | Polynomial graphs are analyzed in calculus using intercepts, slopes, concavity, and end behavior. | ||
| + | |||
| + | ==Resources== | ||
| + | * [https://math.libretexts.org/Courses/Borough_of_Manhattan_Community_College/MAT_206_Precalculus/3%3A_Polynomial_and_Rational_Functions_New/3.4%3A_Graphs_of_Polynomial_Functions Graphs of Polynomial Functions], Mathematics LibreTexts | ||
==References== | ==References== | ||
# Shi, Yongtang, et al. Graph Polynomials. CRC Press, Taylor Et Francis Group, 2017. | # Shi, Yongtang, et al. Graph Polynomials. CRC Press, Taylor Et Francis Group, 2017. | ||
Revision as of 22:54, 8 October 2021
In mathematics, a graph polynomial is a graph invariant whose values are polynomials. Invariants of this type are studied in algebraic graph theory.[1] Important graph polynomials include:
- The characteristic polynomial, based on the graph's adjacency matrix.
- The chromatic polynomial, a polynomial whose values at integer arguments give the number of colorings of the graph with that many colors.
- The dichromatic polynomial, a 2-variable generalization of the chromatic polynomial
- The flow polynomial, a polynomial whose values at integer arguments give the number of nowhere-zero flows with integer flow amounts modulo the argument.
- The (inverse of the) Ihara zeta function, defined as a product of binomial terms corresponding to certain closed walks in a graph.
- The Martin polynomial, used by Pierre Martin to study Euler tours
- The matching polynomials, several different polynomials defined as the generating function of the matchings of a graph.
- The reliability polynomial, a polynomial that describes the probability of remaining connected after independent edge failures
- The Tutte polynomial, a polynomial in two variables that can be defined (after a small change of variables) as the generating function of the numbers of connected components of induced subgraphs of the given graph, parameterized by the number of vertices in the subgraph.
Graphs
A polynomial function in one real variable can be represented by a graph.
-
The graph of the zero polynomial
- f(x) = 0
-
The graph of a degree 0 polynomial
- f(x) = a0, where a0 ≠ 0,
-
The graph of a degree 1 polynomial (or linear function)
- f(x) = a0 + a1x, where a1 ≠ 0,
-
The graph of a degree 2 polynomial
- f(x) = a0 + a1x + a2x2, where a2 ≠ 0
-
The graph of a degree 3 polynomial
- f(x) = a0 + a1x + a2x2 + a3x3, where a3 ≠ 0
-
The graph of any polynomial with degree 2 or greater
- f(x) = a0 + a1x + a2x2 + ⋯ + anxn, where an ≠ 0 and n ≥ 2
A non-constant polynomial function tends to infinity when the variable increases indefinitely (in absolute value). If the degree is higher than one, the graph does not have any asymptote. It has two parabolic branches with vertical direction (one branch for positive x and one for negative x).
Polynomial graphs are analyzed in calculus using intercepts, slopes, concavity, and end behavior.
Resources
- Graphs of Polynomial Functions, Mathematics LibreTexts
References
- Shi, Yongtang, et al. Graph Polynomials. CRC Press, Taylor Et Francis Group, 2017.