Graphs of the Sine and Cosine Functions

$\sin {x}$ (red) and $\sin {\left({\frac {1}{2}}x\right)}$ (green, horizontal stretch of sin(x) by factor of 2). Horizontal stretches/compressions of sine and cosine functions change the period.

$\sin {x}$ (red) and $\sin {\left(2x\right)}$ (green, vertical stretch of sin(x) by factor of 2). Vertical stretches/compressions of sine and cosine functions change the amplitude.

The sine and cosine functions have several distinct characteristics:

• They are periodic functions.
• The domain of each function is $(-\infty ,\infty )$ and the range is [−1,1].
• The graph of $y=\sin {x}$ is symmetric about the origin.
• The graph of $y=\cos {x}$ is symmetric about the y-axis.
• The period of
• Given $y=A\sin {(\omega x)}$ or $y=A\cos {(\omega x)}$ for some real numbers $A$ and $\omega$:

• The amplitude of the function is $|A|$, and represents the maximum distance from the x-axis that the function covers.
• The period (the distance between two peaks or two valleys) is ${\frac {2\pi }{\omega }}$, and represents one full "cycle" of the function.

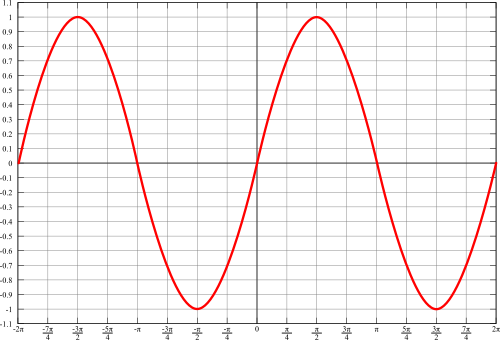
The graph of the sine function looks like this:

Careful analysis of this graph will show that the graph corresponds to the unit circle. x is essentially the degree measure (in radians), while y is the value of the sine function.

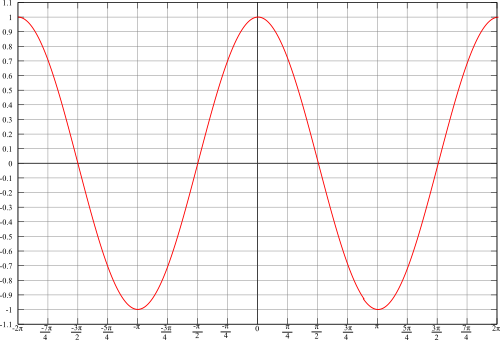
The graph of the cosine function looks like this:

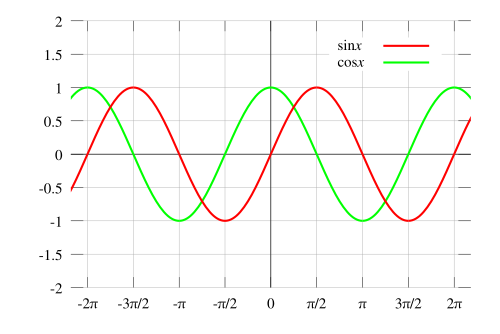
As with the sine function, analysis of the cosine function will show that the graph corresponds to the unit circle. One of the most important differences between the sine and cosine functions is that sine is an odd function (i.e. $\sin(-\theta )=-\sin(\theta )$ while cosine is an even function (i.e. $\cos(-\theta )=\cos(\theta )$ .

Sine and cosine are periodic functions; that is, the above is repeated for preceding and following intervals with length $2\pi$ .

Resources

• Graphs of the Sine and Cosine. Written notes created by Professor Esparza, UTSA.
• Graphs of the Sine and Cosine Functions, Lumen Learning