Difference between revisions of "Absolute Value Functions"
Jump to navigation
Jump to search
| Line 9: | Line 9: | ||
==Resources== | ==Resources== | ||
| + | * [https://math.libretexts.org/Bookshelves/Precalculus/Precalculus_(OpenStax)/01%3A_Functions/1.06%3A_Absolute_Value_Functions Absolute Value Functions], LibreTexts | ||
| + | * [https://www.khanacademy.org/math/algebra/x2f8bb11595b61c86:absolute-value-piecewise-functions/x2f8bb11595b61c86:graphs-of-absolute-value-functions/a/absolute-value-equations-and-graphs-review Absolute Value Graphs Review], Khan Academy | ||
| + | * [https://www.youtube.com/watch?v=sCcsnuwihEw How To Solve Absolute Value Equations], The Organic Chemistry Tutor | ||
| + | * [https://www.youtube.com/watch?v=Tj_tQxtI3gs Solving an Equation with Two Absolute Value Expressions], patrickJMT | ||
Revision as of 12:22, 15 September 2021
Introduction
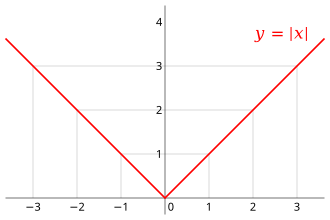
In mathematics, the absolute value or modulus of a real number x, denoted |x|, is the non-negative value of x without regard to its sign. Namely, |x| = x if x is positive, and |x| = −x if x is negative (in which case −x is positive), and |0| = 0. For example, the absolute value of 3 is 3, and the absolute value of −3 is also 3. The absolute value of a number may be thought of as its "distance from zero". The absolute value function f(x) = |a| can be expressed as a piecewise function, where f(x) = a when a ≥ 0, and f(x) = -a when a < 0. We can use this to help us visualize, graph, or solve other absolute value functions.
Here are some examples of absolute value functions:
- . when x ≥ -1, and 5x + 5 < 0 when x < -1. So, when x ≥ -1, and when x < -1.
- . when -2 ≥ x or x ≥ 2. So, when -2 ≥ x or x ≥ 2, and when -2 < x < 2.
Resources
- Absolute Value Functions, LibreTexts
- Absolute Value Graphs Review, Khan Academy
- How To Solve Absolute Value Equations, The Organic Chemistry Tutor
- Solving an Equation with Two Absolute Value Expressions, patrickJMT