Difference between revisions of "Piecewise Linear Function"
Jump to navigation
Jump to search
| Line 1: | Line 1: | ||
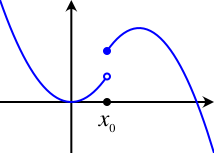
[[Image:Upper_semi.svg|right]]A piecewise function is a function that is given by different expressions on different intervals. The graph of <math>f(x) = \begin{cases} x^2, & \mbox{if}\ x< 2 \\ - \left(x - 3\right)^2 +9, & \mbox{if}\ x\ge2 \end{cases}</math> is shown on the right. The open circle indicates that the point is not included on the graph and the closed circle indicates that the point is included on the graph. So <math>f(2)</math> = 8 not 4. | [[Image:Upper_semi.svg|right]]A piecewise function is a function that is given by different expressions on different intervals. The graph of <math>f(x) = \begin{cases} x^2, & \mbox{if}\ x< 2 \\ - \left(x - 3\right)^2 +9, & \mbox{if}\ x\ge2 \end{cases}</math> is shown on the right. The open circle indicates that the point is not included on the graph and the closed circle indicates that the point is included on the graph. So <math>f(2)</math> = 8 not 4. | ||
| − | |||
| − | |||
Revision as of 16:18, 2 October 2021
A piecewise function is a function that is given by different expressions on different intervals. The graph of is shown on the right. The open circle indicates that the point is not included on the graph and the closed circle indicates that the point is included on the graph. So = 8 not 4.
