Difference between revisions of "Equation of an Ellipse"
(Created page with "* [https://courses.lumenlearning.com/waymakercollegealgebra/chapter/equations-of-ellipses/ Equations of Ellipses], Lumen Learning") |
|||
| Line 1: | Line 1: | ||
| + | == Parametric representation == | ||
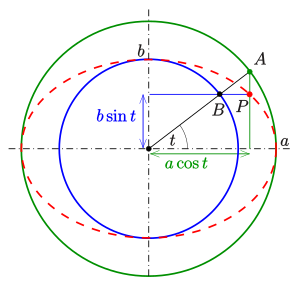
| + | [[File:Elliko-sk.svg|thumb|The construction of points based on the parametric equation and the interpretation of parameter ''t'', which is due to de la Hire]] | ||
| + | |||
| + | ===Standard parametric representation=== | ||
| + | Using trigonometric functions, a parametric representation of the standard ellipse <math>\tfrac{x^2}{a^2}+\tfrac{y^2}{b^2} = 1</math> is: | ||
| + | : <math>(x,\, y) = (a \cos t,\, b \sin t),\ 0 \le t < 2\pi\ .</math> | ||
| + | |||
| + | The parameter ''t'' (called the ''eccentric anomaly'' in astronomy) is not the angle of <math>(x(t),y(t))</math> with the ''x''-axis, but has a geometric meaning due to Philippe de La Hire. | ||
| + | |||
| + | ===Rational representation=== | ||
| + | With the substitution <math display="inline">u = \tan\left(\frac{t}{2}\right)</math> and trigonometric formulae one obtains | ||
| + | :<math>\cos t = \frac{1 - u^2}{u^2 + 1}\ ,\quad \sin t = \frac{2u}{u^2 + 1}</math> | ||
| + | |||
| + | and the ''rational'' parametric equation of an ellipse | ||
| + | : <math>\begin{align} | ||
| + | x(u) &= a\frac{1 - u^2}{u^2 + 1} \\ | ||
| + | y(u) &= \frac{2bu}{u^2 + 1} | ||
| + | \end{align}\;,\quad -\infty < u < \infty\;,</math> | ||
| + | |||
| + | which covers any point of the ellipse <math>\tfrac{x^2}{a^2} + \tfrac{y^2}{b^2} = 1</math> except the left vertex <math>(-a,\, 0)</math>. | ||
| + | |||
| + | For <math>u \in [0,\, 1],</math> this formula represents the right upper quarter of the ellipse moving counter-clockwise with increasing <math>u.</math> The left vertex is the limit <math display="inline">\lim_{u \to \pm \infty} (x(u),\, y(u)) = (-a,\, 0)\;.</math> | ||
| + | |||
| + | |||
| + | ==Resources== | ||
* [https://courses.lumenlearning.com/waymakercollegealgebra/chapter/equations-of-ellipses/ Equations of Ellipses], Lumen Learning | * [https://courses.lumenlearning.com/waymakercollegealgebra/chapter/equations-of-ellipses/ Equations of Ellipses], Lumen Learning | ||
Revision as of 14:34, 18 October 2021
Contents
Parametric representation
Standard parametric representation
Using trigonometric functions, a parametric representation of the standard ellipse is:
The parameter t (called the eccentric anomaly in astronomy) is not the angle of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (x(t),y(t))} with the x-axis, but has a geometric meaning due to Philippe de La Hire.
Rational representation
With the substitution Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\textstyle u = \tan\left(\frac{t}{2}\right)} and trigonometric formulae one obtains
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \cos t = \frac{1 - u^2}{u^2 + 1}\ ,\quad \sin t = \frac{2u}{u^2 + 1}}
and the rational parametric equation of an ellipse
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} x(u) &= a\frac{1 - u^2}{u^2 + 1} \\ y(u) &= \frac{2bu}{u^2 + 1} \end{align}\;,\quad -\infty < u < \infty\;,}
which covers any point of the ellipse Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tfrac{x^2}{a^2} + \tfrac{y^2}{b^2} = 1} except the left vertex Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (-a,\, 0)} .
For Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u \in [0,\, 1],} this formula represents the right upper quarter of the ellipse moving counter-clockwise with increasing Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u.} The left vertex is the limit Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\textstyle \lim_{u \to \pm \infty} (x(u),\, y(u)) = (-a,\, 0)\;.}
Resources
- Equations of Ellipses, Lumen Learning
