Difference between revisions of "Definition of Polygons"
(Created page with "File:Assorted polygons.svg|Assorted polygons|Some polygons of different kinds: open (excluding its boundary), boundary only (excluding interior), closed (including both boun...") |
|||
| Line 1: | Line 1: | ||
| − | [[File:Assorted polygons.svg|Assorted polygons|Some polygons of different kinds: open (excluding its boundary), boundary only (excluding interior), closed (including both boundary and interior), and self-intersecting.]] | + | [[File:Assorted polygons.svg|thumb|Assorted polygons|Some polygons of different kinds: open (excluding its boundary), boundary only (excluding interior), closed (including both boundary and interior), and self-intersecting.]] |
In geometry, a polygon (/ˈpɒlɪɡɒn/) is a plane figure that is described by a finite number of straight line segments connected to form a closed polygonal chain (or polygonal circuit). The bounded plane region, the bounding circuit, or the two together, may be called a polygon. | In geometry, a polygon (/ˈpɒlɪɡɒn/) is a plane figure that is described by a finite number of straight line segments connected to form a closed polygonal chain (or polygonal circuit). The bounded plane region, the bounding circuit, or the two together, may be called a polygon. | ||
| Line 44: | Line 44: | ||
* Rectilinear: the polygon's sides meet at right angles, i.e., all its interior angles are 90 or 270 degrees. | * Rectilinear: the polygon's sides meet at right angles, i.e., all its interior angles are 90 or 270 degrees. | ||
* Monotone with respect to a given line ''L'': every line orthogonal to L intersects the polygon not more than twice. | * Monotone with respect to a given line ''L'': every line orthogonal to L intersects the polygon not more than twice. | ||
| + | |||
| + | == Licensing == | ||
| + | Content obtained and/or adapted from: | ||
| + | * [https://en.wikipedia.org/wiki/Polygon Polygon, Wikipedia] under a CC BY-SA license | ||
Revision as of 18:54, 11 December 2021
In geometry, a polygon (/ˈpɒlɪɡɒn/) is a plane figure that is described by a finite number of straight line segments connected to form a closed polygonal chain (or polygonal circuit). The bounded plane region, the bounding circuit, or the two together, may be called a polygon.
The segments of a polygonal circuit are called its edges or sides. The points where two edges meet are the polygon's vertices (singular: vertex) or corners. The interior of a solid polygon is sometimes called its body. An n-gon is a polygon with n sides; for example, a triangle is a 3-gon.
A simple polygon is one which does not intersect itself. Mathematicians are often concerned only with the bounding polygonal chains of simple polygons and they often define a polygon accordingly. A polygonal boundary may be allowed to cross over itself, creating star polygons and other self-intersecting polygons.
A polygon is a 2-dimensional example of the more general polytope in any number of dimensions. There are many more generalizations of polygons defined for different purposes.
Contents
Etymology
The word polygon derives from the Greek adjective πολύς (polús) 'much', 'many' and γωνία (gōnía) 'corner' or 'angle'. It has been suggested that γόνυ (gónu) 'knee' may be the origin of gon.
Classification
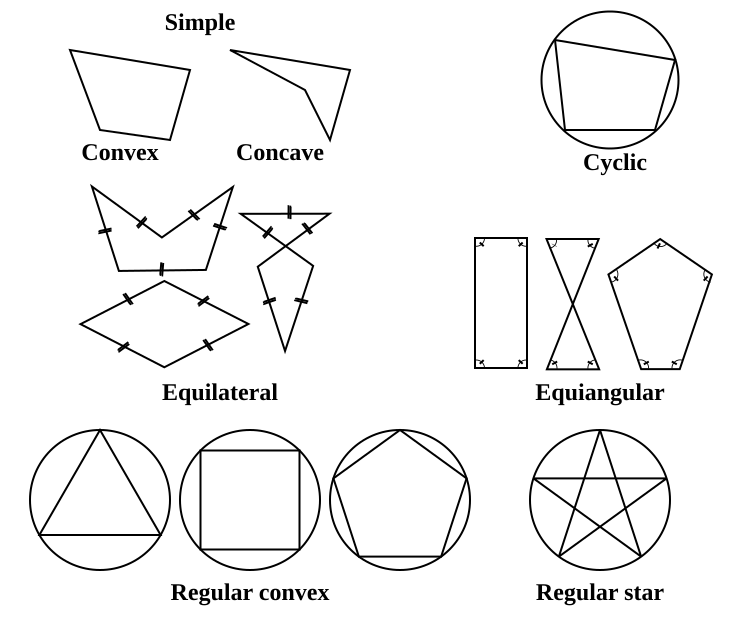 Some different types of polygon
Some different types of polygon
Number of sides
Polygons are primarily classified by the number of sides. See the table below.
Convexity and non-convexity
Polygons may be characterized by their convexity or type of non-convexity:
- Convex: any line drawn through the polygon (and not tangent to an edge or corner) meets its boundary exactly twice. As a consequence, all its interior angles are less than 180°. Equivalently, any line segment with endpoints on the boundary passes through only interior points between its endpoints.
- Non-convex: a line may be found which meets its boundary more than twice. Equivalently, there exists a line segment between two boundary points that passes outside the polygon.
- Simple: the boundary of the polygon does not cross itself. All convex polygons are simple.
- Concave: Non-convex and simple. There is at least one interior angle greater than 180°.
- Star-shaped: the whole interior is visible from at least one point, without crossing any edge. The polygon must be simple, and may be convex or concave. All convex polygons are star-shaped.
- Self-intersecting: the boundary of the polygon crosses itself. The term complex is sometimes used in contrast to simple, but this usage risks confusion with the idea of a complex polygon as one which exists in the complex Hilbert plane consisting of two complex dimensions.
- Star polygon: a polygon which self-intersects in a regular way. A polygon cannot be both a star and star-shaped.
Equality and symmetry
- Equiangular: all corner angles are equal.
- Equilateral: all edges are of the same length.
- Regular: both equilateral and equiangular.
- Cyclic: all corners lie on a single circle, called the circumcircle.
- Tangential: all sides are tangent to an inscribed circle.
- Isogonal or vertex-transitive: all corners lie within the same symmetry orbit. The polygon is also cyclic and equiangular.
- Isotoxal or edge-transitive: all sides lie within the same symmetry orbit. The polygon is also equilateral and tangential.
The property of regularity may be defined in other ways: a polygon is regular if and only if it is both isogonal and isotoxal, or equivalently it is both cyclic and equilateral. A non-convex regular polygon is called a regular star polygon.
Miscellaneous
- Rectilinear: the polygon's sides meet at right angles, i.e., all its interior angles are 90 or 270 degrees.
- Monotone with respect to a given line L: every line orthogonal to L intersects the polygon not more than twice.
Licensing
Content obtained and/or adapted from:
- Polygon, Wikipedia under a CC BY-SA license
