Equation of an Ellipse
Jump to navigation
Jump to search
Contents
Cartesian Equation of an Ellipse
The general equation for an ellipse where its major, or longer, axis is horizontal is : .
Where the major axis is vertical, it is:
- The center is located at .
- The foci are found at a distance of from the centre along the major axis, where .
- The eccentricity of the ellipse can be found from the formula: where e is eccentricity. A higher eccentricity makes the curve appear more 'squashed', whereas an eccentricity of 0 makes the ellipse a circle.
- The directrices are the lines
- The major axis has a length of and the minor one .
- The sum of the distance from each point to each of the foci is .
Parametric representation
Standard parametric representation
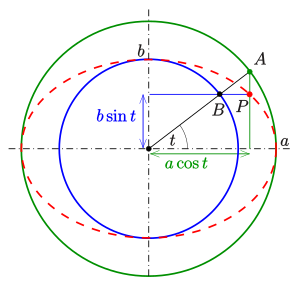
Using trigonometric functions, a parametric representation of the standard ellipse is:
The parameter t (called the eccentric anomaly in astronomy) is not the angle of with the x-axis, but has a geometric meaning due to Philippe de La Hire.
Rational representation
With the substitution and trigonometric formulae one obtains
and the rational parametric equation of an ellipse
which covers any point of the ellipse except the left vertex .
For this formula represents the right upper quarter of the ellipse moving counter-clockwise with increasing The left vertex is the limit
Resources
- Equations of Ellipses, Lumen Learning
Licensing
Content obtained and/or adapted from:
- Ellipse, Wikibooks: Conic Sections under a CC BY-SA license
- Ellipse, Wikipedia under a CC BY-SA license


















![{\displaystyle u\in [0,\,1],}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c61b780db9ac550dd283876e16abe9c2cccdf8c3)

