Derivatives Rates of Change

Average Rate of Change

Rate of Change is used to describe the ratio of a change in one variable compare to a change of another variable

If there is a function $f(x)=y$ then the rate of change of the function $f(x)$ correspond to the rate of change of variable z is

${\frac {\Delta y}{\Delta x}}={\frac {\Delta f(x)}{\Delta x}}$

For example, if there are two points $(1,3)$ and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (2,7)} , then

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\Delta y}{\Delta x}=\frac{\Delta f(x)}{\Delta x}=\frac{7-3}{2-1}=4}

The Rate of Change of a Function at a Point

Consider the formula for average velocity in the $x$ direction, Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\Delta x}{\Delta t}} , where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta x} is the change in $x$ over the time interval Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta t} . This formula gives the average velocity over a period of time, but suppose we want to define the instantaneous velocity. To this end we look at the change in position as the change in time approaches 0. Mathematically this is written as: $\lim _{\Delta t\to 0}{\frac {\Delta x}{\Delta t}}$ , which we abbreviate by the symbol Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{dx}{dt}} . (The idea of this notation is that the letter Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d} denotes change.) Compare the symbol $d$ with Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta} . The idea is that both indicate a difference between two numbers, but Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta} denotes a finite difference while $d$ denotes an infinitesimal difference. Please note that the symbols Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dx} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dt} have no rigorous meaning on their own, since $\lim _{\Delta t\to 0}\Delta t=0$ , and we can't divide by 0.

(Note that the letter Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle s} is often used to denote distance, which would yield Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{ds}{dt}} . The letter $d$ is often avoided in denoting distance due to the potential confusion resulting from the expression Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{dd}{dt}} .)

Definition of Instantaneous Rate of Change (Derivative)

Let Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(x)} be a function. Then $f'(x)=\lim _{h\to 0}{\frac {f(x+h)-f(x)}{h}}$ wherever this limit exists. In this case we say that Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} is differentiable at Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} and its derivative at $x$ is Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f'(x)} .

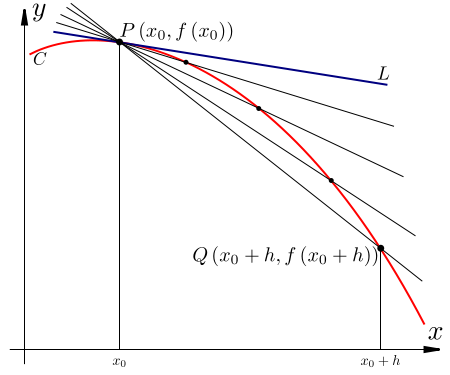
Think of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h } as being the distance between the points $(x_{0},f(x_{0}))$ and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (x_0 + h, f(x_0 + h)) } . Then, the average rate of change between these two points is

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\Delta f(x)}{\Delta x} = \frac{f(x_0+h)-f(x_0)}{(x_0 + h) - x_0} = \frac{f(x_0+h)-f(x_0)}{h}} .

As the distance between the points goes to 0, we approach the instantaneous rate of change (slope) of $f(x)$ at the point Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x = x_0 } . This is how we derive (no pun intended) the definition of the derivative.

Resources

• Derivatives as Rates of Change PowerPoint file created by Dr. Sara Shirinkam, UTSA.

• Derivatives as Rates of Change Worksheet

• Average and Instantaneous Rate of Change of a function over an interval & a point - Calculus by The Organic Chemistry Tutor

• Finding Instantaneous Rates of Change Using Def'n of Derivative by patrickJMT

Licensing

Content obtained and/or adapted from:

• Differentiation Defined, Wikibooks: Calculus/Differentiation under a CC BY-SA license